CINTA作业四

1.证明命题9.1

$$1.\because G\rightarrow H是同构映射，所以G和H是同构的，\\ \because \phi^{-1}也是双射,且G和H是同构的\\ \therefore\phi^{-1}:H\rightarrow G也是同构.\\ 2.\because G\rightarrow H是双射，显然满足一一对应\\ \therefore |G|=|H|\\ 3.\because 同构，\therefore任取a,b\in G,有\phi(a\cdot b)=\phi(a)\circ\phi(b)\\ \because G是阿贝尔群，\therefore\phi(a\cdot b)=\phi(b\cdot a)=\phi(b)\circ\phi(a)\\ \therefore \phi(a)\circ\phi(b)=\phi(b)\circ\phi(a)\\ \therefore H也是阿贝尔群.\\ 4.\because G是循环群，\therefore 存在g是G的生成元\\ \because G\rightarrow G是同构,\\ \therefore\phi(g^2)=\phi(g\cdot g)=\phi(g)\circ\phi(g)=\phi(g)^2\\ \phi(g^n)同理，\therefore\exist \phi(g)为H的生成元，\\ \therefore H也是循环群\\ 5.\because 这是一种双射，一一对应\\ \therefore G的子集G'对应于H的子集H',阶都是n\\ 封闭性：\\设\phi(a),\phi(b)\in H,\\ \therefore a,b\in G,ab\in G\\ \because \phi(a\cdot b)=\phi(a)\circ\phi(b)\\ \therefore\phi(a)\circ\phi(b)\in G \\封闭性得证\\ 结合律:显然满足\\ 单位元：\\ \because a,e\in G,\therefore \phi(a),\phi(e)\in H,\\ \phi(e\cdot a)=\phi(a)=\phi(a)\circ\phi(e)\\ 即\phi(e)是单位元\\ 逆元：\\ \because a,a^{-1}\in G,\therefore \phi(a),\phi(a^{-1})\in H\\ \phi(a\cdot a^{-1})=\phi(e)=\phi(a)\circ\phi(a^{-1})\\ 即存在逆元.$$

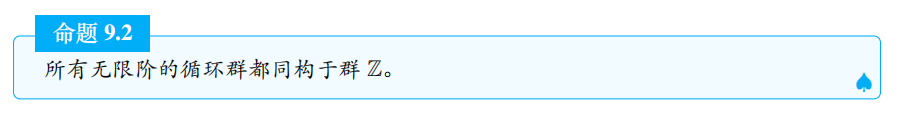
2.给出完整证明

$$设群G是一个无限阶的循环群，g\in G是生成元。\\ 定义\phi:Z_n\rightarrow G 为\phi:n\rightarrow g^n,\\ 则\phi(m+n)=g^{m+n}=g^mg^n=\phi(m)\phi(n).\\ 然后证明\phi是双射:\\ (1)单射：\forall g^a,g^b\in G,总存在a,b\in Z,\\ 若g^a=g^b,则a=b,满足单射。\\ (2)满射：对于\forall g^n\in G,总存在n\in Z\\ 使得\phi(n)=g^n,满足满射。$$